College Algebra
Answer/Discussion to Practice Problems
Tutorial 23A: Quadratic Inequalities
WTAMU > Virtual Math Lab > College Algebra > Tutorial 23A: Quadratic Inequalities
 Answer/Discussion
to 1a
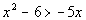
I used the test-point method, but you could also use the
sign graph of
factors method. |
|
*Inv. of sub. 5x is add. 5x
|
|
*Factor
*Set 1st factor = 0 and solve
*Set 2nd factor = 0 and solve
|
-6 and 1 are boundary points. |
Below is a graph that marks off the boundary points -6
and 1 and shows
the three sections that those points have created on the graph.
Note
that open holes were used on those two points since our original
inequality
does not include where it is equal to 0.
Note that the two boundary points create three sections
on the graph:  ,  ,
and  . |
You can choose ANY point in an interval to represent
that interval.
Remember that we are not interested in the actual value that we get,
but
what SIGN (positive or negative) that we get.
Keep in mind that our inequality is  .
Since we are looking for the quadratic expression to be GREATER
THAN 0, that means we need our sign to be POSITIVE.
From the interval ,
I choose to use -7 to test this interval:
(I could have used -10, -25, or -10000 as long as it is in the interval) |
|
*Chose -7 from 1st interval to
plug in for x
|
Since 8 is positive and we are looking for values
that cause our
quadratic expression to be greater than 0 (positive), would be part of the solution. |
From the interval ,
I choose to use 0 to test this interval.
(I could have used -5, -4, or -3 as long as it is in the interval) |
|
*Chose 0 from 2nd interval to plug
in for x
|
Since -6 is negative and we are looking for values
that cause our
expression to be greater than 0 (positive), would not be part of the solution. |
From the interval ,
I choose to use 2 to test this interval.
(I could have used 10, 25, or 10000 as long as it is in the interval) |
|
*Chose 2 from 3rd interval to plug
in for x
|
Since 8 is positive and we are looking for values
that cause our
quadratic expression to be greater than 0 (positive), would be part of the solution. |
Interval notation: 
Graph:
 |
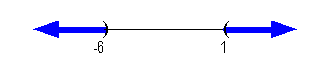*Open intervals indicating all
values less
than -6 or greater than 1
*Visual showing all numbers
less than -6 or
greater than 1
|
Answer/Discussion
to 1b
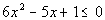
I used the test-point method, but you could also use the sign graph of
factors method.
|
This quadratic inequality is already in standard form. |
|
*Factor
*Set 1st factor = 0 and solve
*Set 2nd factor = 0 and solve
|
1/3 and 1/2 are boundary points. |
Below is a graph that marks off the boundary points,
1/3 and 1/2, and
shows the three sections that those points have created on the
graph.
Note that closed holes were used on those two points since our original
inequality includes where it is equal to 0.
Note that the two boundary points create three sections
on the graph:  ,  , and  . |
You can choose ANY point in an interval to represent
that interval.
Remember that we are not interested in the actual value that we get,
but
what SIGN (positive or negative) that we get.
Keep in mind that our original problem is .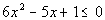
Since we are looking for the quadratic expression to be LESS
THAN OR EQUAL TO 0, that means we need our sign to be NEGATIVE
(OR 0).
From the interval ,
I choose to use 0 to test this interval:
(I could have used -10, -25, or -10000 as long as it is in the interval) |
|
*Chose 0 from 1st interval to plug
in for x
|
Since 1 is positive and we are looking for values
that cause our
quadratic expression to be less than or equal to 0 (negative or
0), would
not be part of the solution. |
From the interval ,
I choose to use 2/5 to test this interval.
(I could have used 5/12 or 3/8 as long as it is in the interval) |
|
*Chose 2/5 from 2nd interval to
plug in for x
|
Since -1/25 is negative and we are looking for
values that cause
our expression to be less than or equal to 0 (negative or
0), would be part of the solution. |
From the interval ,
I choose 1 to use to test this interval.
(I could have used 10, 25, or 10000 as long as it is in the interval) |
|
*Chose 1 from 3rd interval to plug
in for x
|
Since 2 is positive and we are looking for values
that cause our
quadratic expression to be less than or equal to 0 (negative or
0), would not be part of the solution. |
Interval notation:
Graph:
 |
*Closed interval indicating
all values between
1/3 and 1/2, inclusive
*Visual showing all numbers
between 1/3 and
1/2, inclusive
|
Last revised on Dec. 30, 2009 by Kim Seward.
All contents copyright (C) 2002 - 2010, WTAMU and Kim Seward.
All rights reserved.
|
|
