Title
West Texas A&M University - Home
Virtual Math Lab

College Algebra
Answer/Discussion to Practice Problems  
Tutorial 17: Quadratic Equations

WTAMU > Virtual Math Lab > College Algebra > Tutorial 17: Quadratic Equations


 

 

check markAnswer/Discussion to 1a

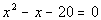
problem 1a

 
Step 1: Simplify each side if needed.

 
This quadratic equation is already simplified.

 
Step 2: Write in standard form, standard form, if needed.

 
This quadratic equation is already in standard form.

 
Step 3: Factor.

 
ad1a1
*Quad. eq. in standard form
*Factor the trinomial

 
Step 4:  Use the Zero-Product Principle

AND

Step 5: Solve for the linear equation(s) set up in step 4. 

 
ad1a2
*Use Zero-Product Principle
*Solve the first linear equation
 
 
 
 
 

*Solve the second linear equation
 

 

 
There are two solutions to this quadratic equation: x = -4 and x = 5.

 


 

 

check markAnswer/Discussion to 1b

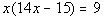
problem 1b

 
Step 1: Simplify each side if needed.

 
ad1b1
*Use Dist. Prop. to clear the (  )

 
Step 2: Write in standard form, standard form, if needed.

 
ad1b2

*Inverse of add. 9 is sub. 9
*Quad. eq. in standard form

 
Step 3: Factor.

 
ad1b3
*Quad. eq. in standard form
*Factor the trinomial

 
Step 4:  Use the Zero-Product Principle

AND

Step 5: Solve for the linear equation(s) set up in step 4. 

 
ad1b4
*Use Zero-Product Principle
*Solve the first linear equation
 
 
 
 
 
 
 
 
 

*Solve the second linear equation
 
 
 
 
 

 

 
There are two solutions to this quadratic equation: x = -3/7 and x = 3/2.

 


 

 

check markAnswer/Discussion to 2a

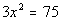
problem 2a

 
Step 1: Write the quadratic equation in the form square root method if needed

AND

Step 2: Apply the square root method.

 
Note how this quadratic equation is not in the form 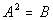square root method to begin with.  The 3 is NOT part of the expression being squared on the left side of the equation.  We can easily write it in the form 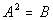square root method by dividing both sides by 3.

 
ad2a1
*Not in the form 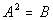square root method
*Inv. of mult. by 3 is div. by 3

*Written in the form 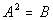square root method

*Apply the sq. root method
*There are 2 solutions

 
Step 3: Solve for the linear equation(s) set up in step 2. 

 
ad2a2
*Sq. root of 25 = 5
 
 
 
 
 

*Neg. sq. root of 25 = -5
 

 
There are two solutions to this quadratic equation: x = 5 and x = -5.

 


 

 

check markAnswer/Discussion to 2b

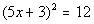
problem 2b

 
Step 1: Write the quadratic equation in the form square root method if needed

AND

Step 2: Apply the square root method.

 
ad2b1
*Written in the form 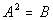square root method

*Apply the sq. root method
*There are 2 solutions

 
Step 3: Solve for the linear equation(s) set up in step 2. 

 
ad2b2
*Sq. root of 12 = 2 sq. root of 3
*Solve for x
 
 
 
 
 
 
 
 
 
 
 

*Neg. sq. root of 12 = -2 sq. root of 3
*Solve for x
 
 
 
 
 

 

 
There are two solutions to this quadratic equation: x = ad2b3  and x = ad2b4.

 


 

 

check markAnswer/Discussion to 3a

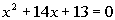
problem 3a

 
Step 1: Make sure that the coefficient on the x squared term is equal to 1.

 
The coefficient of the x squared term is already 1.

 
Step 2:  Isolate the x squared and x terms.

 
Note how the x squared and x terms are not isolated to begin with.  We can easily fix that by moving the constant to the other side of the equation.

 
ad3a1
*Inverse of add. 13 is sub. 13

*x squared and x terms are now isolated
 

 

 
ad3a2
*b is the coefficient of the x term

*Complete the square by taking 1/2 of b and squaring it
 

 

 
ad3a3
*Add constant found above to BOTH sides of the eq.

*This creates a PST on the left side of eq. 

 
Step 4:  Factor the perfect square trinomial (created in step 3) as a binomial squared.

 
ad3a4

*Factor the PST

 
Step 5:  Solve the equation in step 4 by using the square root method.

 
ad3a5
*Written in the form 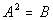square root method
*Apply the sq. root method
*There are 2 solutions
 
 
 
 
 
 
 
 
 

 

 
There are two solutions to this quadratic equation: x = -1  and x = -13.

 


 

 

check markAnswer/Discussion to 3b

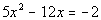
problem 3b

 
Step 1:  Make sure that the coefficient on the x squared term is equal to 1.

 
Note how the coefficient on the x squared term is not 1 to begin with.  We can easily fix that by dividing both sides by that coefficient, which in this case is 5 .

 
ad3b1

*Divide both sides by 5
 

*Coefficient of x squared term is now 1
 

 
Step 2:  Isolate the x squared and x terms.

 
The x squared and x terms are already isolated.

 

 
ad3b2
*b is the coefficient of the x term
 
 

*Complete the square by taking 1/2 of b and squaring it
 
 
 
 

 

 
ad3b3
*Add constant found above to BOTH sides of the eq.
 
 

*This creates a PST on the left side of eq. 
 

 
Step 4:  Factor the perfect square trinomial (created in step 3) as a binomial squared.

 
ad3b4

*Factor the PST

 
Step 5:  Solve the equation in step 4 by using the square root method.

 
ad3b5
*Written in the form 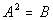square root method
*Apply the sq. root method
*There are 2 solutions
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 

 

 
There are two solutions to this quadratic equation:  x = ad3b6  and x = ad3b7.

 


 

 

check markAnswer/Discussion to 4a

problem 4a

 
Step 1: Simplify each side if needed.

 
This quadratic equation is already simplified.

 
Step 2: Write in standard form, standard form, if needed.

 
This quadratic equation is already in standard form.

 

 
a, the number in front of x squared, is 1.

b, the number in front of x, is 10. 

c, the constant, is 25.
 

Make sure that you keep the sign that is in front of each of these numbers. 

Next we will plug it into the quadratic formula.   Note that we are only plugging in numbers, we don't also plug in the variable.

 
Step 4: Plug the values found in step 3 into the quadratic formula

AND

Step 5: Simplify if possible. 

 
ad4a
*Quadratic formula
 
 

*Plug in values found above for a, b, and c

*Simplify 
 
 
 
 
 
 
 
 
 

 

 


 

check markAnswer/Discussion to 4b

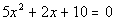
problem 4b

 
Step 1: Simplify each side if needed.

 
This quadratic equation is already simplified.

 
Step 2: Write in standard form standard form if needed.

 
This quadratic equation is already in standard form.

 

 
a, the number in front of x squared, is 5.

b, the number in front of x, is 2. 

c, the constant, is 10.
 

Make sure that you keep the sign that is in front of each of these numbers. 

Next we will plug it into the quadratic formula.   Note that we are only plugging in numbers, we don't also plug in the variable.

 
Step 4: Plug the values found in step 3 into the quadratic formula

AND

Step 5: Simplify if possible. 

 
ad4b
*Quadratic formula
 
 

*Plug in values found above for a, b, and c

*Simplify 
 
 
 
 

*Square root of a negative 1 is i
 
 
 
 
 
 
 

 

 


 

 

check markAnswer/Discussion to 4c

problem 4c

 
Step 1: Simplify each side if needed.

 
This quadratic equation is already simplified.

 
Step 2: Write in standard form standard form if needed.

 
ad4c1

*Inverse of add. 7x and 20 is sub. 7x and 20

*Quad. eq. in standard form

 

 
a, the number in front of x squared, is 3.

b, the number in front of x, is -7. 

c, the constant, is -20.
 

Make sure that you keep the sign that is in front of each of these numbers. 

Next we will plug it into the quadratic formula.   Note that we are only plugging in numbers, we don't also plug in the variable.

 
Step 4: Plug the values found in step 3 into the quadratic formula

AND

Step 5: Simplify if possible. 

 
ad4c2
*Quadratic formula
 
 
 

*Plug in values found above for a, b, and c

*Simplify 
 
 
 
 
 
 
 
 
 
 
 
 
 

 

 


 

 

check markAnswer/Discussion to 5a

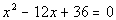
problem 5a

 
Step 1: Simplify each side if needed.

 
This quadratic equation is already simplified.

 
Step 2: Write in standard form, standard form, if needed.

 
This quadratic equation is already in standard form.

 

 
a, the number in front of x squared, is 1.

b, the number in front of x, is -12.

c, the constant, is 36.
 

Make sure that you keep the sign that is in front of each of these numbers. 

 
Step 4: Plug the values found in step 3 into the discriminant, discriminant,

AND

Step 5: Simplify if possible. 

 
ad5a
*Discriminant formula

*Plug in values found above for a, b, and c

*Discriminant

 
Since the discriminant is zero, that means there is only one real number solution.

 


 

 

check markAnswer/Discussion to 5b

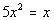
problem 5b

 
Step 1: Simplify each side if needed.

 
This quadratic equation is already simplified.

 
Step 2: Write in standard form, standard form, if needed.

 
ad5b1

*Inverse of add. x is sub. x

*Quad. eq. in standard form

 

 
a, the number in front of x squared, is 5.

b, the number in front of x, is -1.

c, the constant, is 0.
Note that since the constant is missing it is understood to be 0.
 

Make sure that you keep the sign that is in front of each of these numbers. 

 
Step 4: Plug the values found in step 3 into the discriminant, discriminant,

AND

Step 5: Simplify if possible. 

 
ad5b2
*Discriminant formula

*Plug in values found above for a, b, and c

*Discriminant

 
Since the discriminant is a positive number, that means there are two distinct real number solutions.

 


 

check markAnswer/Discussion to 5c

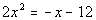
problem 5c

 
Step 1: Simplify each side if needed.

 
This quadratic equation is already simplified.

 
Step 2: Write in standard form, standard form, if needed.

 
ad5c1

*Inverse of sub. x and 12 is add. x and 12

*Quad. eq. in standard form

 

 
a, the number in front of x squared, is 2.

b, the number in front of x, is 1.

c, the constant, is 12.
 

Make sure that you keep the sign that is in front of each of these numbers. 

 
Step 4: Plug the values found in step 3 into the discriminant, discriminant,

AND

Step 5: Simplify if possible. 

 
ad5c2
*Discriminant formula

*Plug in values found above for a, b, and c

*Discriminant

 
Since the discriminant is a negative number, that means there are two distinct complex imaginary solutions.

 

 

buffalo top

 


Last revised on Dec. 16, 2009 by Kim Seward.
All contents copyright (C) 2002 - 2010, WTAMU and Kim Seward. All rights reserved.