College Algebra
Answer/Discussion to Practice Problems
Tutorial 48: Practice Test on Tutorials 42 - 47
Problems 1a - 1b: Graph the following functions. |
Problem 2a: Find the 1) compound amount
AND 2) the compound interest for the given investment and rate.
|
2a. $25000 for 21 years at an annual rate of 3.25%
compounded quarterly.
 Answer: Answer:
P = 25000
r = 3.25% = .0325
t = 21
n = quarterly = 4 times
a year
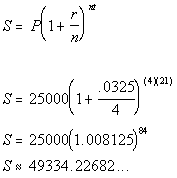
Compound Amount: $49334.23
Compound Interest: $24334.23
|
|
Problem 3a: Find the accumulated value for the given
investment and rate. |
3a. $4525 that is compounded continuously for 12 years
at an interest rate of 9 ½ %.
 Answer: Answer:
P = 4525
r = 9.5% = .095
t = 12

So the accumulated or compound AMOUNT would be
$14148.63.
|
|
Problems 4a - 4b: Express the given logarithmic
equation exponentially. |
Problems 5a - 5b: Express the given
exponential equation in a logarithmic form.
|
Problems 6a - 6b: Evaluate the given log
function without using a calculator. |
Problems 7a - 7b: Graph the following functions. |
7a. 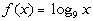
|
7b. 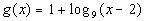
|
Problems 8a - 8b: Evaluate the given
expression without the use of a calculator. |
Problems 9a - 9b: Expand each logarithmic
expression as much as possible. Evaluate without a calculator
where possible. |
Problems
10a - 10b: Condense each logarithmic expression into one
logarithmic
expression. Evaluate without a calculator where possible. |
Problems 11a - 11c: Solve the
given exponential equation. Round your answer to two decimal
places. |
11c. 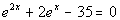
 Answer: Answer:

Since e raised to
a power cannot equal a negative number, there is only one solution, x is approx 1.61.
|
|
Problems 12a - 12b: Solve the
logarithmic equation. Round your answer to two decimal places. |
12b. 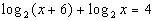
 Answer: Answer:
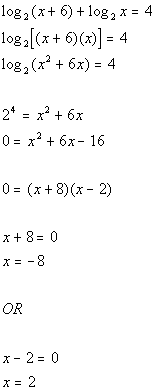
x = 2 is the only solution.
|
|

Last revised on March 25, 2011 by Kim Seward.
All contents copyright (C) 2002 - 2011, WTAMU and Kim Seward.
All rights reserved.
|
|


