Beginning Algebra
Tutorial 11: Simplifying Algebraic Expressions
 Learning Objectives
After completing this tutorial, you should be able to:
- Identify a term, coefficient, constant term, and like terms.
- Combine like terms.
- Simplify an expression using distributive property and combining like
terms.
|
Introduction
In this tutorial we will be looking at various
components of terms.
Then we will move on to adding like terms together. Some of these
concepts are based on ideas that were covered in earlier
tutorials.
A lot of times in math you are using previous knowledge to learn new
concepts.
The trick is to not reinvent the wheel each time, but recognize what
you
have done before and draw on that knowledge to help you work through
the
problems. |
Tutorial
A term is a number, variable or the product of a
number and variable(s).
Examples of terms are  ,  ,  , z |
A coefficient is the numeric factor of your
term.
Here are the coefficients of the terms listed above:
|
Term
|
Coefficient
|
|
|
3
|
|
|
5
|
|
|
2
|
|
z
|
1
|
|
A constant term is a term that contains only a number.
In other
words, there is no variable in a constant term.
Examples of constant terms are 4, 100, and -5. |
Like terms are terms that have the exact same
variables raised to
the exact same exponents.
One example of like terms is  .
Another example is  . |
You can only combine terms that are like terms.
You think
of it as the reverse of the distributive property. It is like counting apples and oranges. You
just count up how
many variables you have the same and write the number in front of
the common variable part. |
 Example
1: Simplify 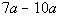 by combining like terms. |
Are there any like terms that we can combine?
It looks like it. Both terms have the same
variable part, a. |
Example
2: Simplify 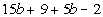 by combining like terms. |
Are there any like terms that we can combine?
It looks like it. Two terms have the same variable
part, b.
The other pair of terms are constant terms that can be combined
together. |
From here on out I will not be
showing the
distributive property step when combining like terms. I
will
go right into adding or subtracting the coefficients of the like terms.
I showed you the distributive property in the above examples to give
you
the thought behind combining like terms. |
Example
3: Simplify the expression  . |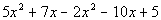
It looks like we have two terms that have an x squared that we can combine and we have two terms that have an x that
we can combine. The poor 5 does not have anything it can combine
with so it will have to stay 5.
Grouping like terms together and combining them we
get: |
|
*Combine the x squared terms
together
and then the x terms together |
Example
4: Simplify the expression  . |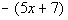
|
*A - outside a ( ) is the same as
times (-1)
*Distribute the (-1) to EVERY term
inside
( )
*Multiply
|
Basically, when you have a negative sign in front of a
( ), like this
example, you can think of it as taking a -1 times the ( ). What
you
end up doing in the end is taking the opposite of every term in the ( ). |
Example
5: Simplify the expression  . |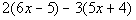
|
*Dist. 2 to EVERY term of 1st
( )
*Dist. -3 to EVERY term of 2nd ( )
*Multiply |
Regrouping and combining like terms we get: |
|
*x is distributed
to the 1st 2 terms
*Reverse Dist. Prop with x
*Subtract
|
Example
6: Write the following as an algebraic
expression
and simplify if possible.
Add 3a + 9 to 7a - 2. |
Basically we will be adding these two expressions
together.
Writing this as an algebraic expression we get: |
|
Regrouping and combining like terms we get: |
Example
7: Write the following as an algebraic
expression
and simplify if possible.
The sum of 5 times a number and 2, subtracted from 12
times a number. |
x is representing the
unknown number.
The sum of 5 times a number and 2 can be written as 5x + 2. From there we need to subtract that from 12x.
Writing this as an algebraic expression we get: |
Practice Problems
�
These are practice problems to help bring you to the
next level.
It will allow you to check and see if you have an understanding of
these
types of problems. Math works just like
anything
else, if you want to get good at it, then you need to practice
it.
Even the best athletes and musicians had help along the way and lots of
practice, practice, practice, to get good at their sport or instrument.
In fact there is no such thing as too much practice. To get the most out of these, you should work the
problem out on
your own and then check your answer by clicking on the link for the
answer/discussion
for that problem. At the link you will find the answer
as well as any steps that went into finding that answer. |
 Practice
Problem 1a: Simplify by combining like terms.
Practice
Problems 2a - 2b: Simplify the expressions.
Practice
Problem 3a: Write the following as an algebraic
expression and
simplify if possible.
Need Extra Help on these Topics?
Last revised on July 26, 2011 by Kim Seward.
All contents copyright (C) 2001 - 2010, WTAMU and Kim Seward. All rights reserved.
|
|
