Title
West Texas A&M University - Home
Virtual Math Lab

Beginning Algebra
Answer/Discussion to Practice Problems  
Tutorial 10: Practice Test on Tutorials 1 - 9

 


Problems 1a - 1c: Replace ? with  < , > , or = . 

 
1a.  -5 ?  5

checkAnswer:

Since -5 is to the left of 5 on the number line, then -5 < 5.

 
1b.  10 ?  5

checkAnswer:

Since 10 is to the right of 5 on the number line, then 10 > 5.

 
1c.  |-5| ? |5|

checkAnswer:

First of all, |-5| = 5.

Next we have |5| = 5.

Since both absolute values equal the same number 5, then  |-5| = |5| .

 
 
Problems 2a - 2b:  Are the following statements true or false?

 
2a.  - 4  >  4

checkAnswer:

Since - 4 is to the left of 4 on the number line, then - 4  <  4. 

So, the above statement is false.

 
2b.  - 4  <  - 4

checkAnswer:

Since - 4 is the same number as - 4 and the statement includes where the two numbers are equal to each other, then this statement is true.

 
 
Problems 3a - 3c:  Write each sentence as a mathematical statement.

 
3a.  5 is not equal to -5.

checkAnswer:

Reading it left to right we get:

5 is not equal to -5
ad3a

 
3b.  -2 is less than or equal to 0.

checkAnswer:

Reading it left to right we get:

-2 is less than or equal to 0
-2  <  0

 
3c.  7 is greater than 0.

checkAnswer:

Reading it left to right we get:

7 is greater than 0
7  >  0

 
 
Problems 4a - 4f :  List the elements of the following set that are also elements of the given set. {-½, 0, 5, 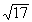problem 4a, problem 4b}

 
4a.  Natural numbers 

checkAnswer:

The numbers in the given set that are also natural numbers are

{5, problem 4a}

 
4b.  Whole numbers 

checkAnswer:

The numbers in the given set that are also whole numbers are

{0, 5, problem 4a}

 
4c.  Integers 

checkAnswer:
 

The numbers in the given set that are also integers are

{0, 5, problem 4a}

 
4d.  Rational numbers 

checkAnswer:
 

The numbers in the given set that are also rational numbers are

{-1/2, 0, 5, problem 4a}

 
4e.  Irrational numbers 

checkAnswer:

The number in the given set that is also an irrational number is

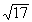{problem 4b}

 
4f.  Real numbers 

checkAnswer:

The numbers in the given set that are also real numbers are

{-½, 0, 5, 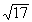problem 4a, problem 4b}

 
 
Problems 5a:  Write the number as a product of primes.

 
5a.  90

checkAnswer:

ad5a

 
 
Problems 6a:  Write the fraction in lowest terms.

 
6a. problem 6a

checkAnswer:

ad6a

 
 
Problems 7a - 7d:  Perform the following operations.  Write answers in the lowest terms.

 
7a. problem 7a

checkAnswer:

ad7a

 
7b. problem 7b

checkAnswer:

ad7b

 
7c. problem 7c

checkAnswer:

ad7c

 
7d. problem 7d

checkAnswer:

ad7d

 
 
Problems 8a - 8b:   Evaluate.

 
8a. problem 8a

checkAnswer:

ad8a

 
8b. problem 8b

checkAnswer:

ad8b

 
 
Problems 9a - 9b:  Write each phrase as an algebraic expression.  Let x represent the unknown number.

 
9a.  The quotient of 7 and a number.

checkAnswer:

The quotient of 7 and a number
ad9a

 
9b.  10 less than 2 times a number.

checkAnswer:

10 less than 2 times a number
ad9b

 
 
Problems 10a - 10b:  Write each sentence as an equation.  Let x represent the unknown number.

 
10a.  The sum of 2 and 10 times a number is the same as 30.

checkAnswer:

The sum of 2 and 10 times a number is the same as 30
ad10a

 
10b.  The product of 5 and a number is 2/3.

checkAnswer:

The product of 5 and a number is 2/3
ad10b

 
 
Problems 11a - 11c:  Add.

 
11a.  -9 + (-10)

checkAnswer:

-9 + (-10) = -19

 
11b. problem 11b

checkAnswer:

ad11b

 
11c.  6.5 + (-1.2) + (-3.1)

checkAnswer:

6.5 + (-1.2) + (-3.1) = 
5.3 + (-3.1) = 
2.2

 
 
Problems 12a - 12b: Simplify.

 
12a.  -(-17)

checkAnswer:

-(-17) = 17

 
12b. problem 12b

checkAnswer:

ad12b

 
 
Problems 13a - 13b: Subtract.

 
13a.  -15 - (-3)

checkAnswer:

-15 - (-3) = 
-15 + 3  =
-12

 
13b.  -1.5 - 2.5

checkAnswer:

-1.5 - 2.5 = 
-1.5 + (-2.5) =
- 4

 
 
Problems 14a - 14c:  Multiply.

 
14a.  (-5)(-12)

checkAnswer:

(-5)(-12) = 60

 
14b.  (3)(-5)(2)

checkAnswer:

(3)(-5)(2) = 
(-15)(2) =
-30

 
14c.  (-15)(0)

checkAnswer:

(-15)(0) = 0

 
 
Problems 15a - 15c: Divide.

 
15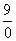a. problem 15a

checkAnswer:

ad15a

 
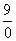15b. problem 15b

checkAnswer:

problem 15b is undefined.

 
15c. problem 15c

checkAnswer:

ad15c

 
 
Problems 16a - 16b:   Simplify.

 
16a. problem 16a

checkAnswer:

ad16a

 
16b. problem 16b

checkAnswer:

ad16b

 
 
Problems 17a - 17b:   Evaluate the expression.

 
17a. problem 17a 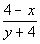  when x = 3 and y = -3.

checkAnswer:

ad17a

 
17b. problem 17b 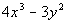 when x = 2 and y = -2

checkAnswer:

ad17b

 
 
Problems 18a - 18b: Decide whether the given number is a solution of the given equation.

 
18a.  Is 1 a solution to 3x - 1 = 4?

checkAnswer:

ad18a

Is 1 a solution? 

Since we got a FALSE statement (2 does not equal 4), then 1 is not a solution.

 
18b.  Is -3 a solution to 7 - x = 2x + 16?

checkAnswer:

ad18b

Is -3 a solution? 

Since we got a TRUE statement (10 does equal 10), then -3 is a solution.

 
 
Problem 19a:  Use a commutative property to write an  equivalent expression.

 
19a.  3a + 2b

checkAnswer:
 

Using the commutative property of addition (where changing the order of a sum does not change the value of it), we get

3a + 2b = 2b + 3a

 
 
Problem 20a:  Use an associative property to write an  equivalent expression.

 
20a.  8(xy)

checkAnswer:

Using the associative property of multiplication (where changing the grouping of a product does not change the value of it), we get 

8(xy) = (8x)y

 
 
Problems 21a - 21b:  Use the distributive property to find the product.

 
21a.    -5(a - 7)

checkAnswer:

ad21a

 
21b.  8(2x + 3y +4z)

checkAnswer:

ad21b

 
 
Problems 22a - 22b: Write the opposite (additive inverse) and the reciprocal (multiplicative inverse) of each number.

 
22a.  -10

checkAnswer:

The opposite of -10 is 10, since -10 + 10 = 0.

The reciprocal of -10 is -1/10, since -10(-1/10) = 1.

 
22b. 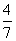problem 22b

checkAnswer:

The opposite of 4/7 is - 4/7, since 4/7 + (- 4/7) = 0.

The reciprocal of 4/7 is 7/4, since (4/7)(7/4) = 1.

 
 
Problem 23a - 23c:   The bar graph below shows the profit a cd store made over the months of September through December of last year.   Use the graph to answer questions 23a - 23c.

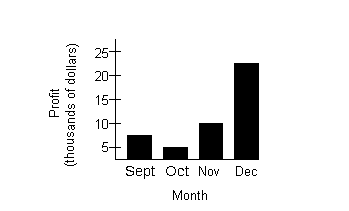
problem 1

 
 
23a.  About how much was the profit in December? 

checkAnswer:

The bar that associates with December is the fourth bar from the left.  The top of that bar is in between 20 and 25 on the vertical axis.  A good approximation is 22.

The profit in December is about $22000.
 
 

23b.  Which month had the lowest profit?

checkAnswer:

It looks like October had the lowest profit.
 
 

23c.  What is the sum of the profits of October and November? 

checkAnswer:

The bar that associates with October is the second bar from the left.  The top of that bar matches with 5 on the vertical axis.

The bar that associates with November is the third bar from the left.  The top of that bar matches with 10 on the vertical axis.

The sum of the profits of October and November would be 5,000 + 10,000 = $15,000.

 
 
Problems 24a - 24b: The line graph below shows last week's high temperatures in Fahrenheit.   Use the graph to answer questions 24a - 24b.

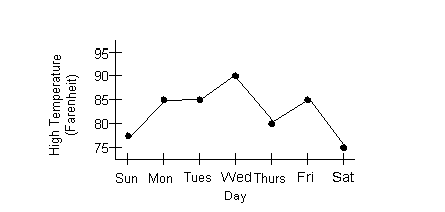
problem 2

 
24a.  How much was Monday's high temperature? 

checkAnswer:

The point that matches with Monday on the horizontal axis also matches 85 on the vertical axis. 

Monday's high temperature was 85 degrees Fahrenheit.
 

24b.  Which day had the highest high temperature? 

checkAnswer:

It looks like Wednesday had the highest high temperature.

 

 

buffalo top

 


Last revised on July 25, 2011 by Kim Seward.
All contents copyright (C) 2001 - 2010, WTAMU and Kim Seward. All rights reserved.